


Next: Selection Rules and Available
Up: The Aufbau Principal, Kramers
Previous: From the Hydrogenic: The
An atom which has a valance electron in an extremely high principal quantum
number state is in a Rydberg state. These states are called circular
states for the following reason. (We use the simpler hydrogen case due
to the fact that it is not a terrible approximation to hydrogenic atoms.)
The radial equation (see the compendium on the hydrogen atom) was found
to be simplifiable to,
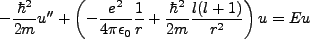 |
(2.1) |
With,
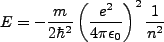 |
(2.2) |
and
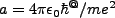 , we can rewrite this equation as,
, we can rewrite this equation as,
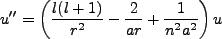 |
(2.3) |
We then consider that (using integration by parts and knowing that the infinite limit of the radial component must be zero)
Algebraic exercise yields Kramer's relation,
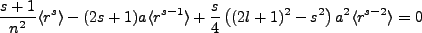 |
(2.5) |
When we set 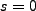 we find,
we find,
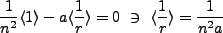 |
(2.6) |
Thus setting 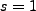 yields,
yields,
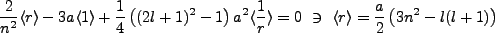 |
(2.7) |
When we use the states such that  and
and 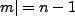 , i.e. the maximum allowed, we have
, i.e. the maximum allowed, we have
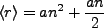 |
(2.8) |
For very large n, this approximates the classical result for circular orbits,
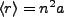 ,
thus the name circular Rydberg states.
,
thus the name circular Rydberg states.



Next: Selection Rules and Available
Up: The Aufbau Principal, Kramers
Previous: From the Hydrogenic: The
tim jones
2007-04-09