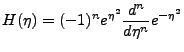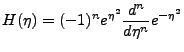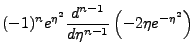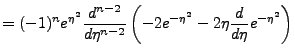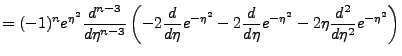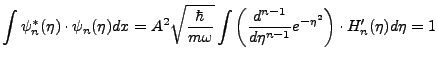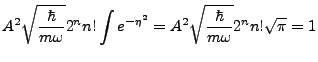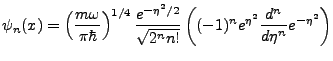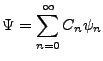Normalization of wave function

The solution we demonstrated is called a Hermite polynomial,

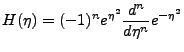$$H(\eta)=(-1)^ne^{\eta^2}\frac{d^n}{d\eta^n}e^{-\eta^2}$$ (2.7)

Properties of this function can be found with repeated activation of the derivatives,

$$H_n(\eta)= (-1)^n e^{\eta^2} \frac{d^{n-1}}{d\eta^{n-1}}\left(-2\eta e^{-\eta^2}\right)$$

$$=(-1)^ne^{\eta^2}\frac{d^{n-2}}{d\eta^{n-2}}\left(-2e^{-\eta^2}-2\eta \frac{d}{d\eta}e^{-\eta^2}\right)$$

$$=(-1)^ne^{\eta^2}\frac{d^{n-3}}{d\eta^{n-3}}\left(-2\frac{d}{d\et... ...-\eta^2}-2\frac{d}{d\eta}e^{-\eta^2}-2\eta\frac{d^2}{d\eta^2}e^{-\eta^2}\right)$$ (2.8)

A continuation of this activation would thus find,

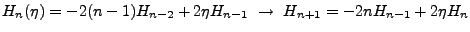$$H_n(\eta)=-2(n-1)H_{n-2} + 2\eta H_{n-1} \ \rightarrow \ H_{n+1}=-2nH_{n-1}+2\eta H_n$$ (2.9)

This implies that,

$$\frac{d}{d\eta}H_n(\eta) =2\eta(-1)^n e^{\eta^2}\frac{d^n}{d\eta^n}e^{-\eta^2} + (-1)^ne^{\eta^2}\frac{d^{n+1}}{d\eta^{n+1}}e^{-\eta^2}$$

$$=2\eta H_n(\eta) - H_{n+1}(\eta) = 2nH_{n-1}(\eta)$$ (2.10)

The normalization equation is, via integration by parts,

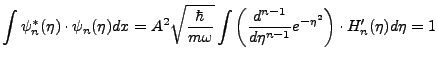$$\int \psi^*_n(\eta) \cdot \psi_n(\eta) dx=A^2\sqrt{\frac{\hbar}{m... ...}\int\left(\frac{d^{n-1}}{d\eta^{n-1}}e^{-\eta^2}\right)\cdot H'_n(\eta)d\eta=1$$ (2.11)

Use of Equation 2.10 and repeated integration by parts will diminish the stand-alone derivative and the Hermite polynomial until we have,

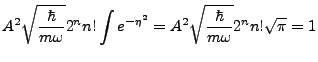$$A^2\sqrt{\frac{\hbar}{m\omega}}2^nn!\int e^{-\eta^2}=A^2 \sqrt{\frac{\hbar}{m\omega}}2^n n!\sqrt{\pi} = 1$$ (2.12)

Thus we have

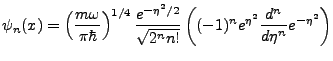$$\psi_n(x)=\left(\frac{m\omega}{\pi \hbar}\right)^{1/4}\frac{e^{-\... ...2/2}}{\sqrt{2^nn!}}\left((-1)^n e^{\eta^2}\frac{d^n}{d\eta^n}e^{-\eta^2}\right)$$ (2.13)

Where a most general solution is

$$\Psi = \sum_{n=0}^{\infty} C_n \psi_n$$

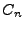Here $C_n$ are coefficients which can only be determined when the specific physical situation is given.