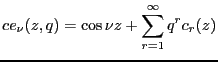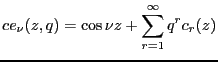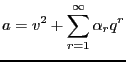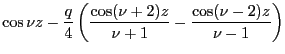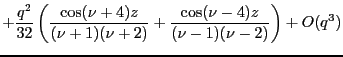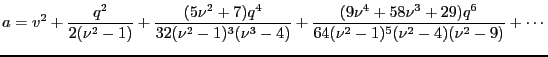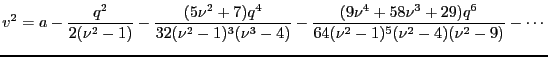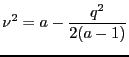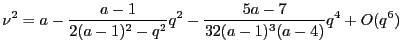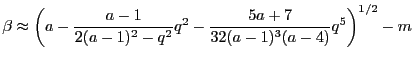Fractional order

Now we suppose solutions of the form

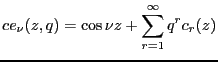$$ce_{\nu}(z,q)=\cos \nu z + \sum_{r=1}^{\infty} q^r c_r(z)$$ (5.5)

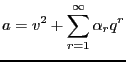$$a = v^2 + \sum_{r=1}^{\infty} \alpha_r q^r$$ (5.6)

Quoting again our references [4,3], the above procedure may be applied to find,

$$ce_{\nu}(z,q) = \cos \nu z -\frac{q}{4}\left(\frac{\cos(\nu + 2)z}{\nu +1} -\frac{\cos(\nu -2)z}{\nu -1}\right)$$

$$+\frac{q^2}{32}\left(\frac{\cos(\nu + 4)z}{(\nu + 1)(\nu +2)} + \frac{\cos(\nu -4)z}{(\nu -1)(\nu -2)}\right) + O(q^3)$$ (5.7)

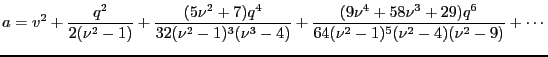$$a=v^2 + \frac{q^2}{2(\nu^2 -1)} + \frac{(5\nu^2 + 7)q^4}{32(\nu^2... ...} + \frac{(9\nu^4 + 58 \nu^3 + 29)q^6}{64(\nu^2-1)^5(\nu^2 -4)(\nu^2-9)}+\cdots$$ (5.8)

The latter can be rewritten,

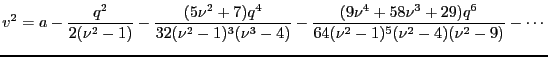$$v^2=a - \frac{q^2}{2(\nu^2 -1)} - \frac{(5\nu^2 + 7)q^4}{32(\nu^2... ...} - \frac{(9\nu^4 + 58 \nu^3 + 29)q^6}{64(\nu^2-1)^5(\nu^2 -4)(\nu^2-9)}-\cdots$$ (5.9)

A first approximation is 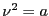$\nu^2 = a$ . Putting this into the $q^2$ coefficient gives a second approximation,

$$\nu^2 =a-\frac{q^2}{2(a-1)}$$

And repeating the process gives

$$\nu^2 =a -\frac{a-1}{2(a-1)^2 -q^2}q^2 -\frac{5a-7}{32(a-1)^3(a-4)}q^4 + O(q^6)$$

Finally we note that 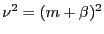$\nu^2 = (m+\beta)^2$ (the integral and fractional component), we have the approximation

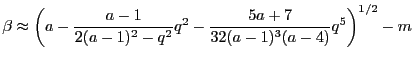$$\beta \approx \left(a -\frac{a-1}{2(a-1)^2 -q^2}q^2 - \frac{5a+7}{32(a-1)^3(a-4)}q^5\right)^{1/2} - m$$ (5.10)

The cosine functions have sine equivalents which we have not included for the sake of brevity. These formulations are not uncommon in the literature, though for obvious reasons the previous derivations were presented in fuller context as they seem to be the preferred method of dealing with the Mathieu equation. But alas, after presenting so many ways of looking at Mathieu's equation, like Pandora's box, last out is hope.