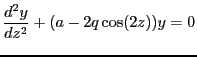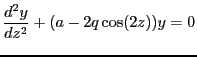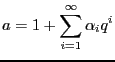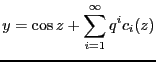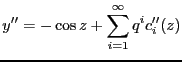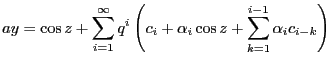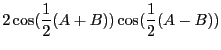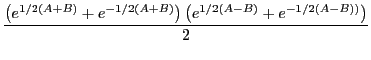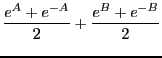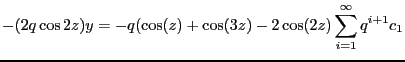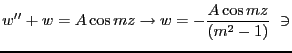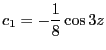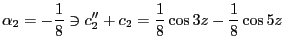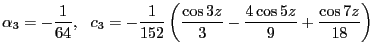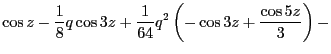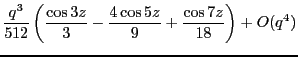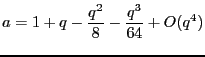Next: Fractional order
Up: Technical details
Previous: Technical details
We write the Mathieu equation as
And consider the case when 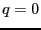 and write
and write 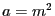 and have solutions
and have solutions
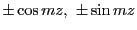 . We then
suppose that the case when q is nonzero can be taken into
account as a series based on this initial solution.
Let
. We then
suppose that the case when q is nonzero can be taken into
account as a series based on this initial solution.
Let
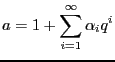 |
(5.1) |
Then we suppose that
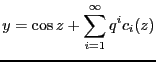 |
(5.2) |
We determine the nature of the 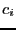 functions as follows. Plugging
our solution into the Mathieu equation, we get
functions as follows. Plugging
our solution into the Mathieu equation, we get
Using the identity
Coefficents are matched:
The particular solution corresponding to
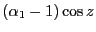 is
is
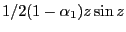 which is not bounded, thus we require
that
which is not bounded, thus we require
that
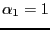 such that
such that
The arguments presented before imply that
Following [4], we write
and so we find the c functions can be represted by ``cosine-elliptic'' function,
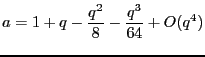 |
(5.4) |



Next: Fractional order
Up: Technical details
Previous: Technical details
tim jones
2008-07-07