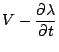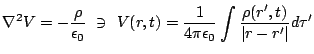Next: Quantization of an electromagnetic
Up: A Brief Introduction to
Previous: Potential Equations Maxwell's Equation.
Equations 1.1,1.2 are not unique. Suppose 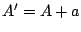 and
and 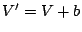 , we
do not violate Maxwell's equations if
and
Typically one redefines
, we
do not violate Maxwell's equations if
and
Typically one redefines
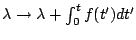 , returning the general gauge transform,
, returning the general gauge transform,
Two common gauges are the Coulomb and Lorenz. The Coulomb gauge has us take 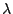 so that
so that
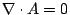 .
Thus Equation 1.1 simplifies to a harmonic equation:
Unfortunately this does not do much to simplify the defining equation for the vector potential,
The Lorenz gauge has us chose
.
Thus Equation 1.1 simplifies to a harmonic equation:
Unfortunately this does not do much to simplify the defining equation for the vector potential,
The Lorenz gauge has us chose
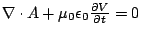 . Define the d'Alembertian
as
. Define the d'Alembertian
as
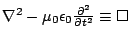 . Then the Lorenz gauge reduces the potential equations
to
. Then the Lorenz gauge reduces the potential equations
to



Next: Quantization of an electromagnetic
Up: A Brief Introduction to
Previous: Potential Equations Maxwell's Equation.
Timothy Jones
2006-05-30